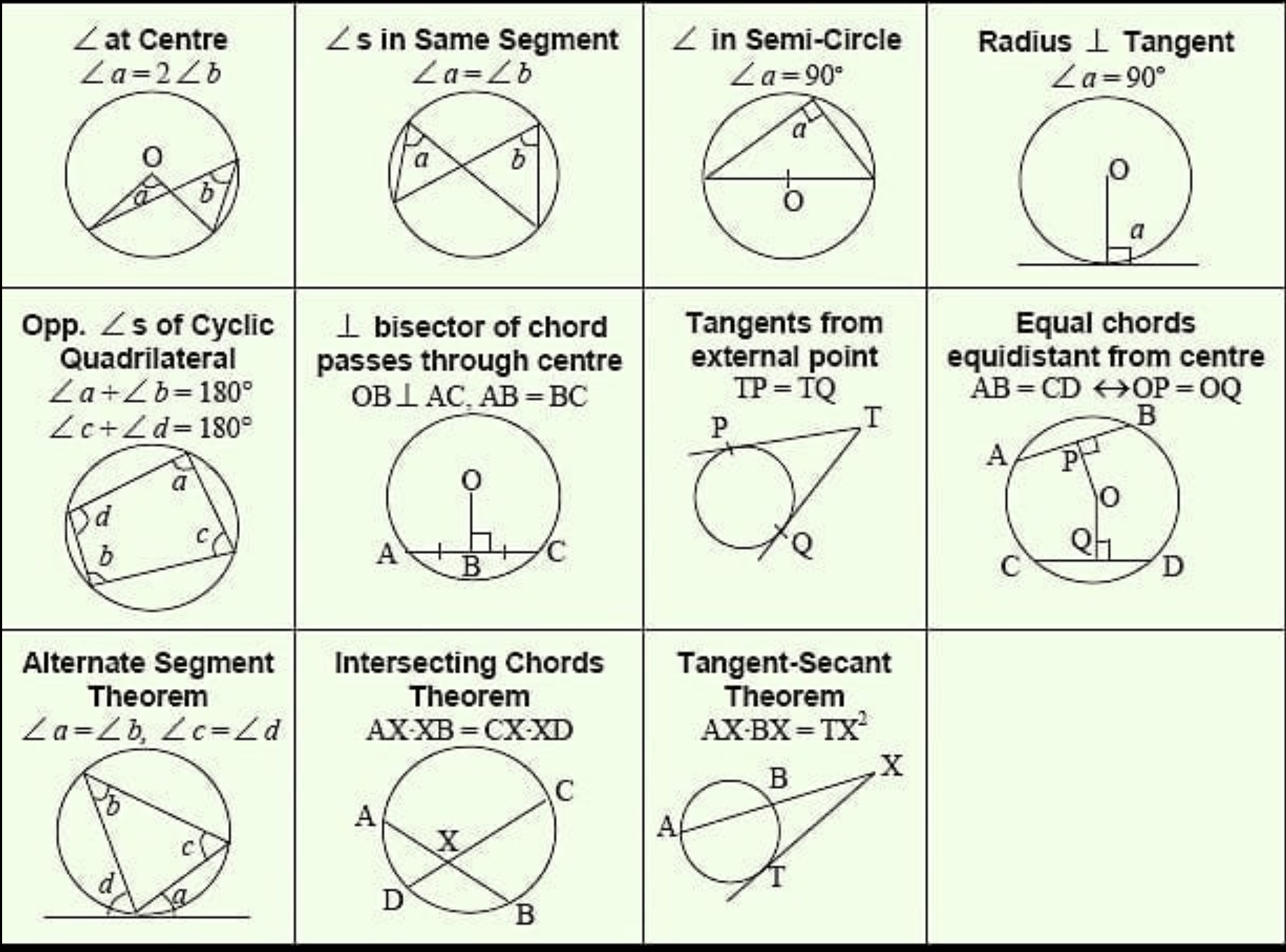
The unit circle is an essential concept in trigonometry, and understanding the tangent rules in unit circle can significantly enhance your mathematical prowess. As you delve into the world of trigonometry, you will discover how the unit circle serves as a powerful tool for visualizing and solving trigonometric problems. With its center at the origin of the coordinate plane and a radius of one, the unit circle provides a unique perspective on the relationships between angles and the trigonometric functions of sine, cosine, and tangent.
In this article, we will explore the tangent rules in unit circle, offering a detailed and structured approach to mastering this fundamental concept. Covering a range of topics, from the basic definition of the unit circle to the practical applications of tangent rules, our guide aims to provide you with the insights needed to excel in your studies. By breaking down complex ideas into manageable sections, we'll ensure that you gain a comprehensive understanding of how tangent rules function within the unit circle.
Designed with students in mind, this article is crafted to be both informative and engaging. We will utilize a variety of teaching methods, including clear explanations, illustrative diagrams, and practical examples, to help you grasp the intricacies of tangent rules in unit circle. Whether you're preparing for an exam or simply looking to deepen your knowledge of trigonometry, this guide will serve as a valuable resource on your educational journey.
Table of Contents
- Definition of the Unit Circle
- Understanding Tangent Rules
- Relationship Between Tangent and Other Trigonometric Functions
- Tangent Rules in Unit Circle Explained
- Applications of Tangent Rules in Unit Circle
- Graphical Representation of Tangent on the Unit Circle
- Calculating Tangent Values Using the Unit Circle
- Common Mistakes and How to Avoid Them
- Advanced Concepts Related to Tangent Rules
- Real-life Examples of Tangent Rules
- Historical Perspective on Tangent Rules
- Frequently Asked Questions
- Conclusion
Definition of the Unit Circle
The unit circle is a fundamental concept in trigonometry, representing a circle with a radius of one unit centered at the origin of a coordinate plane. It is the perfect tool for understanding and visualizing the relationships between angles and various trigonometric functions. The unit circle allows students to explore the properties of sine, cosine, and tangent through an intuitive geometric approach.
In the unit circle, angles are measured in radians, and each point on the circle corresponds to an angle formed with the positive x-axis. The coordinates of these points are directly related to the cosine and sine values of the respective angles. This connection between geometric and algebraic representations makes the unit circle a powerful tool in solving trigonometric problems.
By understanding the unit circle, students can easily determine the values of trigonometric functions for any given angle. This understanding also lays the foundation for more advanced concepts, such as the tangent rules in unit circle, which we will explore in detail in this article.
Understanding Tangent Rules
Tangent is one of the three primary trigonometric functions, alongside sine and cosine. In the context of the unit circle, the tangent of an angle is defined as the ratio of the sine and cosine values of that angle. Mathematically, this is expressed as:
- Tangent (θ) = Sine (θ) / Cosine (θ)
Understanding this definition is crucial for grasping the tangent rules in unit circle. These rules govern how tangent behaves in relation to angles and other trigonometric functions. By mastering these rules, students can efficiently solve trigonometric equations and tackle complex problems with ease.
One key aspect of tangent rules is their periodicity. Like sine and cosine, tangent is a periodic function, repeating its pattern every π radians. This periodic nature allows for predictions and calculations of tangent values across different quadrants of the unit circle. In addition, tangent is an odd function, meaning that its value is the negative of the tangent of the negative angle. These properties play an essential role in understanding the behavior of tangent on the unit circle.
Relationship Between Tangent and Other Trigonometric Functions
The relationship between tangent and the other trigonometric functions—sine and cosine—is foundational to understanding tangent rules in unit circle. As previously mentioned, tangent is defined as the ratio of sine to cosine. This interdependence is crucial for solving trigonometric problems and establishing the connections between these functions.
Each point on the unit circle corresponds to an angle and has coordinates (cosine(θ), sine(θ)). These coordinates serve as the building blocks for calculating tangent values. The tangent of an angle is simply the y-coordinate (sine) divided by the x-coordinate (cosine). This relationship highlights the interconnectedness of trigonometric functions and the importance of the unit circle in visualizing these connections.
Understanding these relationships also helps students identify patterns and symmetries within the unit circle. For example, the signs of sine, cosine, and tangent values change as the angle moves through different quadrants. By recognizing these patterns, students can predict the behavior of tangent and other trigonometric functions without relying on memorization.
Tangent Rules in Unit Circle Explained
The tangent rules in unit circle are a set of guidelines and principles that govern the behavior of the tangent function when applied to angles on the unit circle. These rules are essential for solving trigonometric problems and understanding the relationships between angles and their corresponding tangent values.
One of the primary tangent rules is the periodicity of the tangent function. As previously mentioned, tangent is a periodic function with a period of π radians. This means that for any angle θ, the tangent value will repeat every π radians. This periodic nature allows students to predict tangent values for angles beyond the standard range of 0 to 2π radians.
Another important rule is the symmetry of the tangent function. Tangent is an odd function, meaning that it is symmetric with respect to the origin. Mathematically, this is expressed as:
- Tangent(−θ) = −Tangent(θ)
This symmetry allows students to quickly determine the tangent of negative angles by simply negating the tangent value of the positive angle.
Additionally, the tangent rules in unit circle highlight the undefined nature of tangent at certain angles. Specifically, tangent is undefined at angles where the cosine value is zero, such as π/2 and 3π/2 radians. At these angles, the division by zero occurs, leading to undefined tangent values. Recognizing these undefined points is crucial for solving trigonometric equations and avoiding errors.
Applications of Tangent Rules in Unit Circle
The tangent rules in unit circle have numerous practical applications in mathematics and beyond. By understanding these rules, students can effectively solve trigonometric equations and tackle a wide range of problems in various fields.
One common application of tangent rules is in the field of physics, where trigonometric functions are used to model waveforms and oscillations. The periodic nature of tangent makes it an ideal tool for representing repeating patterns and cycles in physical systems.
In engineering, tangent rules are used to analyze and design mechanical systems, such as gears and pulleys. The ability to calculate tangent values allows engineers to determine angles and forces within these systems, ensuring optimal performance and efficiency.
In addition to these fields, tangent rules are also used in computer graphics, where they help create realistic images and animations. The mathematical precision of the unit circle allows graphic designers to accurately model shapes and movements, resulting in visually stunning digital content.
By mastering the tangent rules in unit circle, students can unlock a wealth of applications and opportunities in various disciplines, enhancing their problem-solving skills and expanding their knowledge of trigonometry.
Graphical Representation of Tangent on the Unit Circle
The graphical representation of tangent on the unit circle provides a visual understanding of how tangent values change as angles vary. By observing the unit circle, students can see the relationship between angles and their corresponding tangent values, aiding in the comprehension of tangent rules in unit circle.
On the unit circle, the tangent of an angle is represented by the length of the line segment that is perpendicular to the radius at the angle and intersects the tangent line of the unit circle. This line segment extends from the point where the angle intersects the unit circle to the tangent line, visually illustrating the tangent value.
As the angle increases, the tangent value changes, reflecting the periodic and symmetric nature of the function. By analyzing these graphical representations, students can identify patterns and symmetries within the unit circle, enhancing their understanding of tangent rules.
In addition to the unit circle, students can also visualize tangent values using graphs of the tangent function. These graphs display the characteristic shape of the tangent curve, with periodic peaks and troughs reflecting the function's behavior.
Calculating Tangent Values Using the Unit Circle
Calculating tangent values using the unit circle is a straightforward process that relies on the relationship between sine and cosine. By understanding this relationship, students can quickly determine tangent values for any given angle.
To calculate the tangent of an angle, first identify the coordinates of the point on the unit circle corresponding to the angle. These coordinates represent the cosine and sine values of the angle. The tangent value is then calculated by dividing the sine value by the cosine value:
- Tangent(θ) = Sine(θ) / Cosine(θ)
This method allows students to efficiently calculate tangent values without relying on memorized values or complex calculations. By practicing this technique, students can develop a strong understanding of tangent rules and improve their problem-solving skills.
In addition to calculating tangent values, students can also use the unit circle to verify the periodicity and symmetry of the tangent function. By observing the repeating patterns and symmetries within the unit circle, students can gain a deeper understanding of tangent rules and their applications.
Common Mistakes and How to Avoid Them
When working with tangent rules in unit circle, students may encounter several common mistakes that can lead to errors in calculations and problem-solving. By recognizing these mistakes and learning how to avoid them, students can improve their understanding of tangent rules and enhance their mathematical skills.
One common mistake is failing to account for the periodicity of the tangent function. Students may mistakenly assume that tangent values are unique for each angle, leading to incorrect calculations. To avoid this mistake, students should remember that tangent is a periodic function with a period of π radians, and use this knowledge to predict tangent values for angles beyond the standard range.
Another mistake is neglecting the symmetry of the tangent function. Students may overlook the fact that tangent is an odd function, leading to incorrect calculations for negative angles. To avoid this mistake, students should remember that Tangent(−θ) = −Tangent(θ), and use this relationship to quickly determine tangent values for negative angles.
Finally, students may encounter errors when dealing with undefined tangent values. At angles where the cosine value is zero, such as π/2 and 3π/2 radians, tangent is undefined. To avoid mistakes, students should identify these points and account for them in their calculations.
Advanced Concepts Related to Tangent Rules
Once students have mastered the basic tangent rules in unit circle, they can explore more advanced concepts that build on this foundation. These concepts provide deeper insights into the behavior of tangent and other trigonometric functions, expanding students' understanding of trigonometry.
One advanced concept is the use of inverse tangent functions. Inverse tangent, also known as arctangent, allows students to determine the angle corresponding to a given tangent value. This concept is particularly useful in solving trigonometric equations and finding angles in real-world applications.
Another advanced concept is the use of tangent in complex numbers. By extending the unit circle to the complex plane, students can explore the relationships between tangent and complex numbers, leading to new insights and applications in fields such as engineering and physics.
Finally, students can explore the use of tangent in calculus, where it plays a crucial role in differentiating and integrating trigonometric functions. By understanding the derivative and integral of tangent, students can tackle complex calculus problems and deepen their knowledge of mathematical analysis.
Real-life Examples of Tangent Rules
The tangent rules in unit circle have numerous real-life applications, demonstrating the practical significance of these mathematical concepts. By understanding these applications, students can appreciate the relevance of trigonometry in everyday life and various fields.
One real-life example is the use of tangent in navigation and surveying. By calculating tangent values, surveyors can determine distances and angles between points on a map, ensuring accurate measurements and efficient navigation.
In the field of architecture, tangent rules are used to design and construct buildings with precise angles and proportions. By applying trigonometric principles, architects can create structures that are both aesthetically pleasing and structurally sound.
In sports, tangent rules are used to analyze and improve athletic performance. By understanding the angles and forces involved in various sports, coaches and athletes can optimize their techniques and achieve better results.
These real-life examples highlight the importance of tangent rules in unit circle and their wide-ranging applications, inspiring students to explore the possibilities of trigonometry in their own lives.
Historical Perspective on Tangent Rules
The concept of tangent and its rules have a rich historical background, tracing back to ancient civilizations that first explored the relationships between angles and trigonometric functions. By understanding this history, students can gain a deeper appreciation for the development of mathematical concepts and their enduring significance.
In ancient Greece, mathematicians such as Hipparchus and Ptolemy laid the groundwork for trigonometry by studying the properties of angles and their relationships to circles. These early pioneers developed the first trigonometric tables and explored the connections between angles and their corresponding functions.
The study of tangent and other trigonometric functions continued to evolve during the Islamic Golden Age, with scholars such as Al-Khwarizmi and Al-Battani making significant contributions to the field. These mathematicians expanded the understanding of trigonometry and developed new methods for calculating tangent values.
In the Renaissance, European mathematicians such as Johannes Kepler and Isaac Newton further advanced the study of trigonometry, exploring the applications of tangent and other functions in physics and astronomy. Their work laid the foundation for modern trigonometry and its applications in science and engineering.
This historical perspective highlights the enduring significance of tangent rules in unit circle and their role in the development of mathematics as a whole.
Frequently Asked Questions
- What is the unit circle?
The unit circle is a circle with a radius of one unit, centered at the origin of a coordinate plane. It is used in trigonometry to visualize the relationships between angles and trigonometric functions.
- How is tangent defined on the unit circle?
Tangent is defined as the ratio of the sine and cosine values of an angle. On the unit circle, tangent is calculated by dividing the y-coordinate (sine) by the x-coordinate (cosine) of the point corresponding to the angle.
- What are the tangent rules in unit circle?
The tangent rules in unit circle include the periodicity, symmetry, and undefined nature of tangent. These rules govern the behavior of tangent values for angles on the unit circle.
- What are some common mistakes when working with tangent rules?
Common mistakes include neglecting the periodicity and symmetry of tangent, as well as encountering undefined tangent values at certain angles. Recognizing these mistakes can help students avoid errors in calculations.
- How are tangent rules used in real-life applications?
Tangent rules are used in navigation, surveying, architecture, sports, and many other fields. They help calculate angles, distances, and forces, demonstrating the practical significance of trigonometry.
- What is the historical significance of tangent rules?
The concept of tangent and its rules have a rich historical background, with contributions from ancient Greek, Islamic, and Renaissance mathematicians. These developments laid the foundation for modern trigonometry and its applications.
Conclusion
In conclusion, mastering the tangent rules in unit circle is an essential step in understanding trigonometry and its wide-ranging applications. By exploring the fundamental concepts, relationships, and properties of tangent, students can enhance their mathematical skills and tackle complex problems with confidence.
This article has provided a comprehensive guide to tangent rules, covering topics such as the definition of the unit circle, the relationship between tangent and other trigonometric functions, and the applications of tangent rules in various fields. By following this guide and practicing the techniques outlined, students can develop a strong foundation in trigonometry and excel in their studies.
As you continue your mathematical journey, remember that the tangent rules in unit circle are just one piece of the puzzle. By exploring other trigonometric functions and concepts, you can further expand your knowledge and unlock new opportunities in mathematics and beyond.
For further reading, you can explore more about trigonometry on Khan Academy's Trigonometry Section.
Article Recommendations